Take the square root of both sides y=√9−x^2 Now write it as y=±√9−x2 '~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~ Calculate and plot a series of points using first the positive version of this equation then repeat using the negative side We get the following graph for x^2 y^2 = 9 9 Example 4 y = (x − 1) 2 Note the brackets in this example they make a big difference!Sketch the graph of the parametric equations x = cos2t, y = cost 1 for t in 0, π Solution We again start by making a table of values in Figure 1022 (a), then plot the points (x, y) on the Cartesian plane in Figure 1022 (b) The curves in Examples 1021 and 1022 are portions of the same parabola (y 1)2 x = 1

Circles
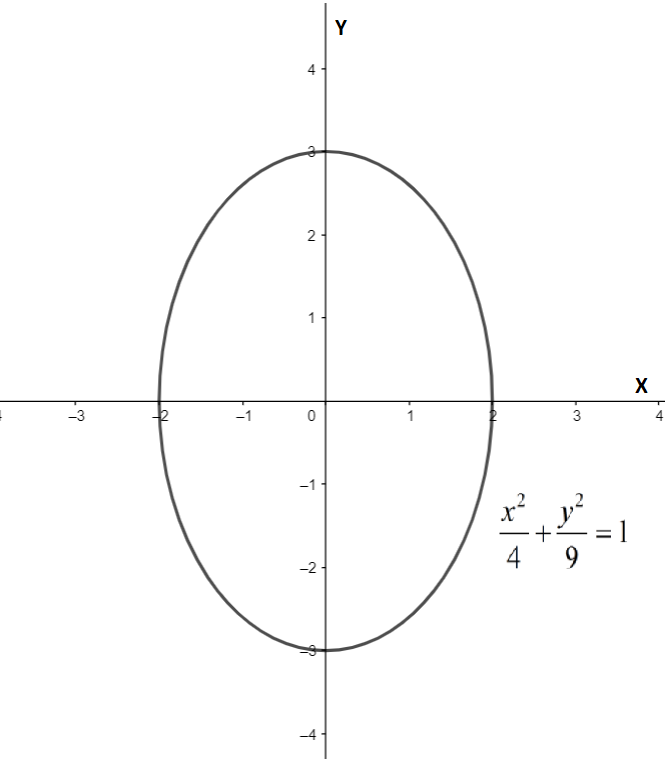
X^2/4 y^2/9=1 graph
X^2/4 y^2/9=1 graph-X^ {2}2\left (x\right)=y3 Subtract 3 from both sides x^ {2}2x=y3 Multiply 2 and 1 to get 2 x^ {2}2x1=y31 Divide 2, the coefficient of the x term, by 2 to get 1 Then add the square of 1 to both sides of the equation This step makes the left hand side of the equation a perfect squareX^2 2 y^2 = 1 Natural Language;



Quiz Chapter 10
Y x2 4, y 0, x 4, Graph b Finding the boundaries and y 00 implies x2 4 0 so x 2 x 2 or x 2 From the graph we see that is our boundary at a The value is a solution to the equation above but it is not bounding the area (Here 's why the graph is an important tool to helpFor different values of the constants \(k\) and \(h\text{}\) Such variations are called transformations of the graph Subsection Vertical Shifts Figure232 shows the graphs of \(f (x) = x^2 4\text{,}\) \(g(x) = x^2 4\text{,}\) and the basic parabola, \(y = x^2\text{}\) By comparing tables of values, we can see exactly how the graphs of \(f\) and \(g\) are related to the basic parabolaAnswer to Solved The graph of the equation x^2/16 y^2/9 = 1 Math;
State its yintercept, domain, and rangeCircle on a Graph Let us put a circle of radius 5 on a graph Now let's work out exactly where all the points are We make a rightangled triangle And then use Pythagoras x 2 y 2 = 5 2 There are an infinite number of those points, here are some examples2x2y=4 Geometric figure Straight Line Slope = 1 xintercept = 2/1 = 0000 yintercept = 2/1 = 0000 Rearrange Rearrange the equation by subtracting what is to the right of the
Example 3 Graph the solution for the linear inequality 2x y ≥ 4 Solution Step 1 First graph 2x y = 4 Since the line graph for 2x y = 4 does not go through the origin (0,0), check that point in the linear inequality Step 2 Step 3 Since the point (0,0) is not in the solution set, the halfplane containing (0,0) is not in the setGraph the parabola, y =x^21 by finding the turning point and using a table to find values for x and yPiece of cake Unlock StepbyStep Natural Language Math Input
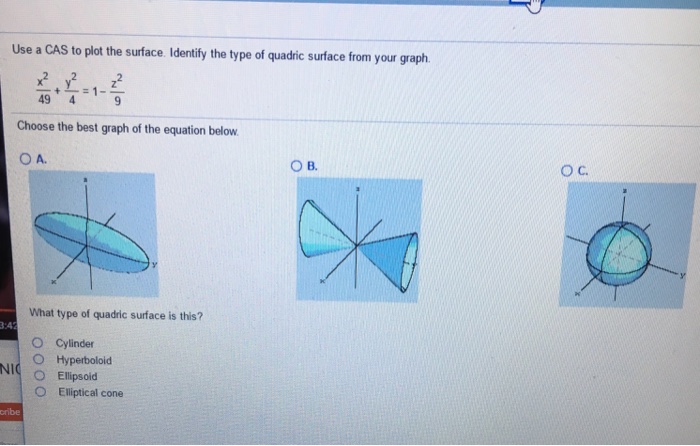



Solved Use A Cas To Plot The Surface Identify The Type Of Chegg Com




Circles
Graph y=1/4x2 y = 1 4 x 2 y = 1 4 x 2 Rewrite in slopeintercept form Tap for more steps The slopeintercept form is y = m x b y = m x b, where m m is the slope and b b is the yintercept y = m x b y = m x b Reorder terms y = 1 4 xBecause there are 2 ellipsoid graphs to choose from, we look at the major axis in the function and pick the graph with the corresponding major axis x axis radius = 1, y axis radius = (sqrt(1/4))^2 z axis radius = (sqrt(1/9))^2 We see the major axis is the x axis, and the corresponding graph is VII This is graph VIIAll equations of the form ax^{2}bxc=0 can be solved using the quadratic formula \frac{b±\sqrt{b^{2}4ac}}{2a} The quadratic formula gives two solutions, one when ± is addition and one when it is subtraction



Quiz Chapter 10



1
Graph the ellipse and its foci x^2/9 y^2/4=1 standard forms of ellipse (xh)^2/a^2(yk)^2/b^2=1 (horizontal major axis),a>b (yk)^2/a^2(xh)^2/b^2=1 (vertical major axis),a>b given ellipse has horizontal major axis center(0,0) a^2=9 a=3 b^2=4 b=2 c=sqrt(a^2b^2)=sqrt(94)=sqrt(5)=224 foci=(224,0),(224,0) see graph of given ellipse below Answer a 16) Elliptic cone For exercises 17 28, rewrite the given equation of the quadric surface in standard form Identify the surface 17) − x 2 36 y 2 36 z 2 = 9 Answer − x 2 9 y 2 1 4 z 2 1 4 = 1, hyperboloid of one sheet with the x axis as its axis of symmetry 18) − 4 x 2 25 y 2 z 2 = 100Example y≤2x1 1 The inequality already has "y" on the left and everything else on the right, so no need to rearrange 2 Plot y=2x1 (as a solid line because y≤ includes equal to) 3 Shade the area below (because y is less than or equal to)




Solve Ellipse And Hyperbola Step By Step Math Problem Solver
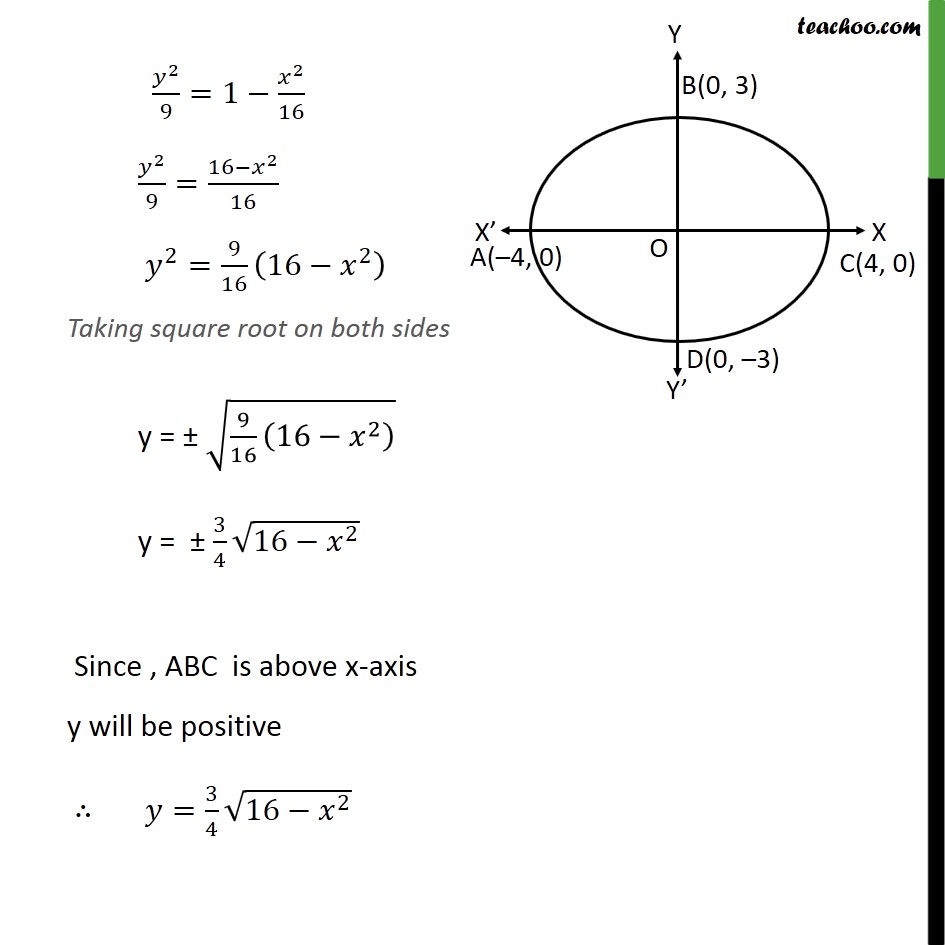



Ex 8 1 4 Find Area Bounded By Ellipse X2 16 Y2 9 1
Ellipsoids are the graphs of equations of the form ax 2 by 2 cz 2 = p 2, where a, b, and c are all positive In particular, a sphere is a very special ellipsoid for which a, b, and c are all equal Plot the graph of x 2 y 2 z 2 = 4 in your worksheet in Cartesian coordinates Then choose different coefficients in the equation, and plot aGraph (x1)^2 (y2)^2=9 (x − 1)2 (y 2)2 = 9 ( x 1) 2 ( y 2) 2 = 9 This is the form of a circle Use this form to determine the center and radius of the circle (x−h)2 (y−k)2 = r2 ( x h) 2 ( y k) 2 = r 2 Match the values in this circle to those of the standard formDescription Function Grapher is a full featured Graphing Utility that supports graphing up to 5 functions together You can also save your work as a URL (website link) Usage To plot a function just type it into the function box



Algebra 2 Ch 9 Solutions Key Ch 9 Solutions Key Pdf Peninsula



Exercises 3 Sketch The Graph Of The Equation 1 Fr Gauthmath
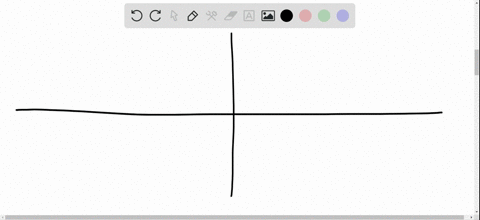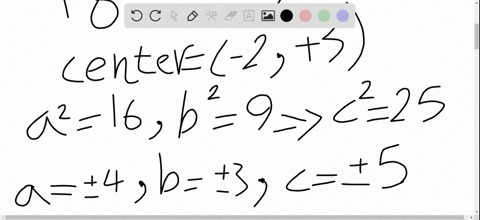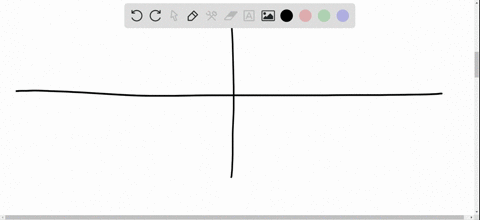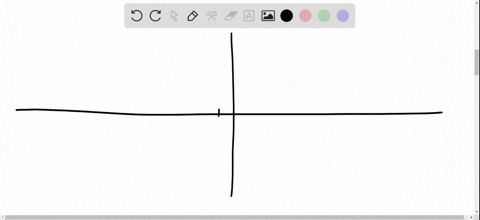
The center is ( 2, 1 ) Since a = 5 is associated with x 2, the major axis is horizontal The vertices are on a horizontal line 5 units to the left and right of the center at ( – 3, 1 ) and ( 7, 1 ) The endpoints of the minor axis are on the vertical line 2 units below and above the center at ( 2, – 1 ) and ( 2, 3 ) The domain is – 3, 7 The range is – 1, 3Extended Keyboard Examples Upload Random Compute answers using Wolfram's breakthrough technology & knowledgebase, relied on by millions of students & professionals For math, science, nutrition, history, geography, engineering, mathematics, linguistics, sports, finance, musicSin (x)cos (y)=05 2x−3y=1 cos (x^2)=y (x−3) (x3)=y^2 y=x^2 If you don't include an equals sign, it will assume you mean " =0 " It has not been well tested, so have fun with it, but don't trust it If it gives you problems, let me know Note it may take a few seconds to finish, because it has to do lots of calculations




260 Precalculus And Algebra Videos Absolute Value Equations Quadratic Equation Precalculus



Find The Foci Of The Graph Of X 2 4 Y 2 9 1 Mathskey Com
40 Vertical x = 53 Horizontal y =0 4 /__/9 pointsThe graph of S(r) = a' is given below y 4 t'r Nwaua 3 2 1Algebra questions and answers; 3 y > 2 A Graph 1 Image Not Shown B Graph 2 Image Not Shown C Graph 3 Image Not Shown Graph the solutions to the inequality 4 x (underscore >) 4 A Graph 1 Image Not Shown B Graph 2 Image Not Shown C Graph 3 Image Not Shown Plz help me, i am terrible in math, and hurry plz!



How To Construct The Graph Of X 2 Y 2 9 Quora




Rd Sharma Solutions For Class 8 Chapter 9 Linear Equation In One Variable Download Free Pdf
The graph of f x =1 2 (1 4) x2 4 f x =1 2 (1 4) x2 4 is shifted downward 4 4 units, and then shifted left 2 2 units, stretched vertically by a factor of 4, 4, and reflected about the xaxis What is the equation of the new function, g (x)?13 Surface 24x 24y2 9z = 35;Algebra > Quadraticrelationsandconicsections> SOLUTION sketch the graph of each ellipse 1x^2/9y^2/4=1 2x^2/9y^2=1 3x^2y^2/4=1 4y^2/4x^2/25=1 5y^2/9X^2/16=1 6x^2/25y^2=1 7x^2y^2/9=1 8x^2y^2/25=1 9x^2/9y^2=1 Log On




Section 11 6 Conic Sections Ppt Video Online Download
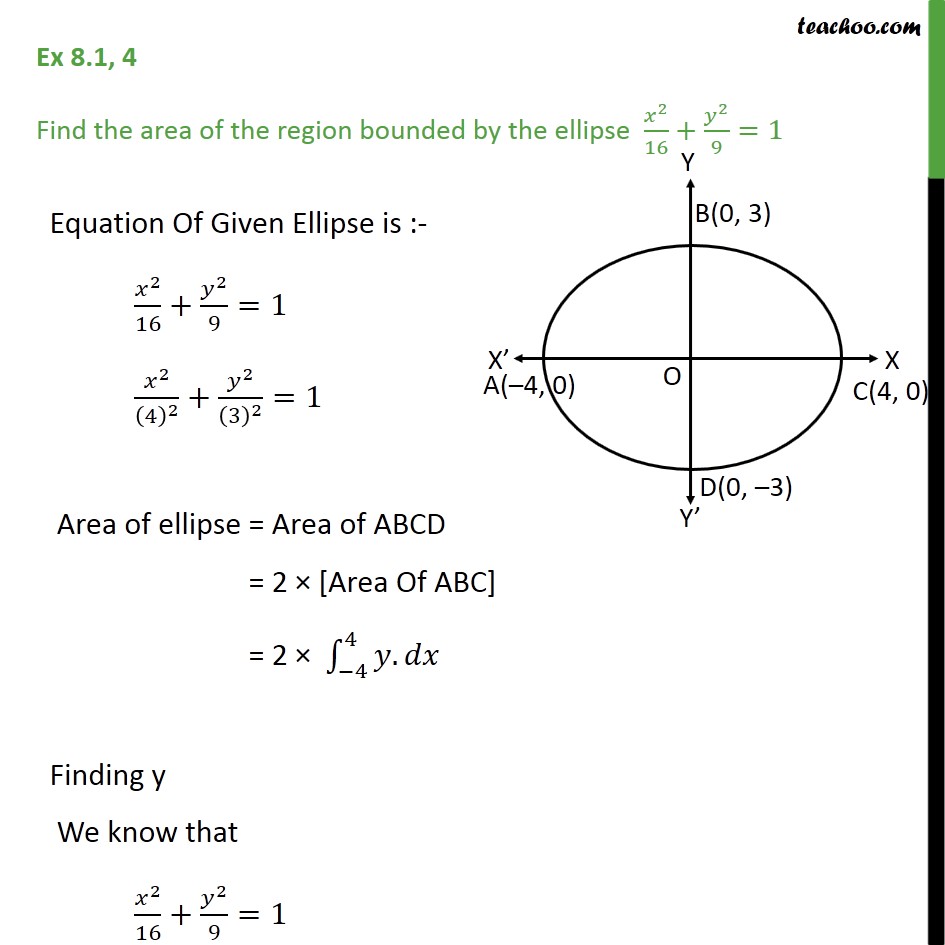



Ex 8 1 4 Find Area Bounded By Ellipse X2 16 Y2 9 1
Divide 2, the coefficient of the x term, by 2 to get 1 Then add the square of 1 to both sides of the equation This step makes the left hand side of the equation a perfect squareSteps to graph x^2 y^2 = 4The graph of the equation x^2/16 y^2/9 = 1 represents a hyperbola



Sd308 Org



Draw A Rough Sketch Of The Graph Of The Curve X 2 4 Y 2 9 1 Sarthaks Econnect Largest Online Education Community
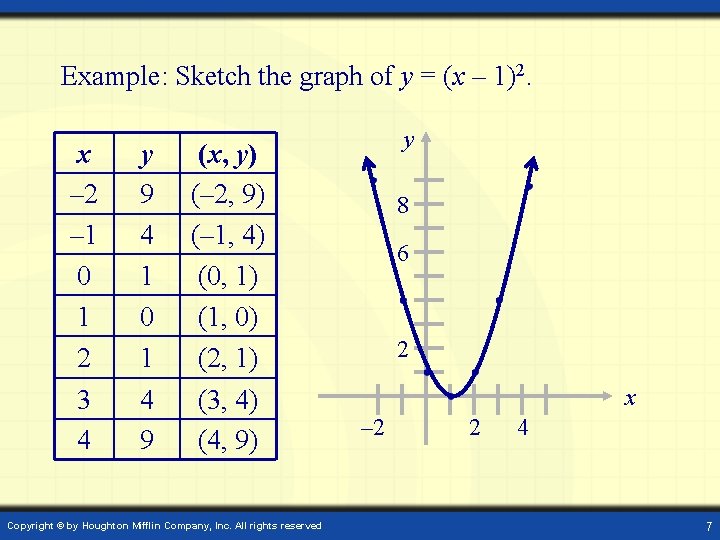
We will use 1 and 4 for x If x = 1, y = 2(1) 6 = 4 if x = 4, y = 2(4) 6 = 2 Thus, two solutions of the equation are (1, 4) and (4, 2) Next, we graph these ordered pairs and draw a straight line through the points as shown in the figure We use arrowheads to show that the line extends infinitely far in both directionsJovannyz0725 jovannyz0725 Mathematics High School answered Which is the graph of x^2/9 y^2/4 = 1 2 See answers AdvertisementIf we think about y = (x − 1) 2 for a while, we realize the yvalue will always be positive, except at x = 1 (where y will equal 0) Before sketching, I will check another (easy) point to make sure I have the curve in the right place



Solved Sketch The Graph Of Each Hyperbola Determine The Foci And The Equations Of The Asymptotes Frac Y 2 2 4 Frac X 1 2 9 1
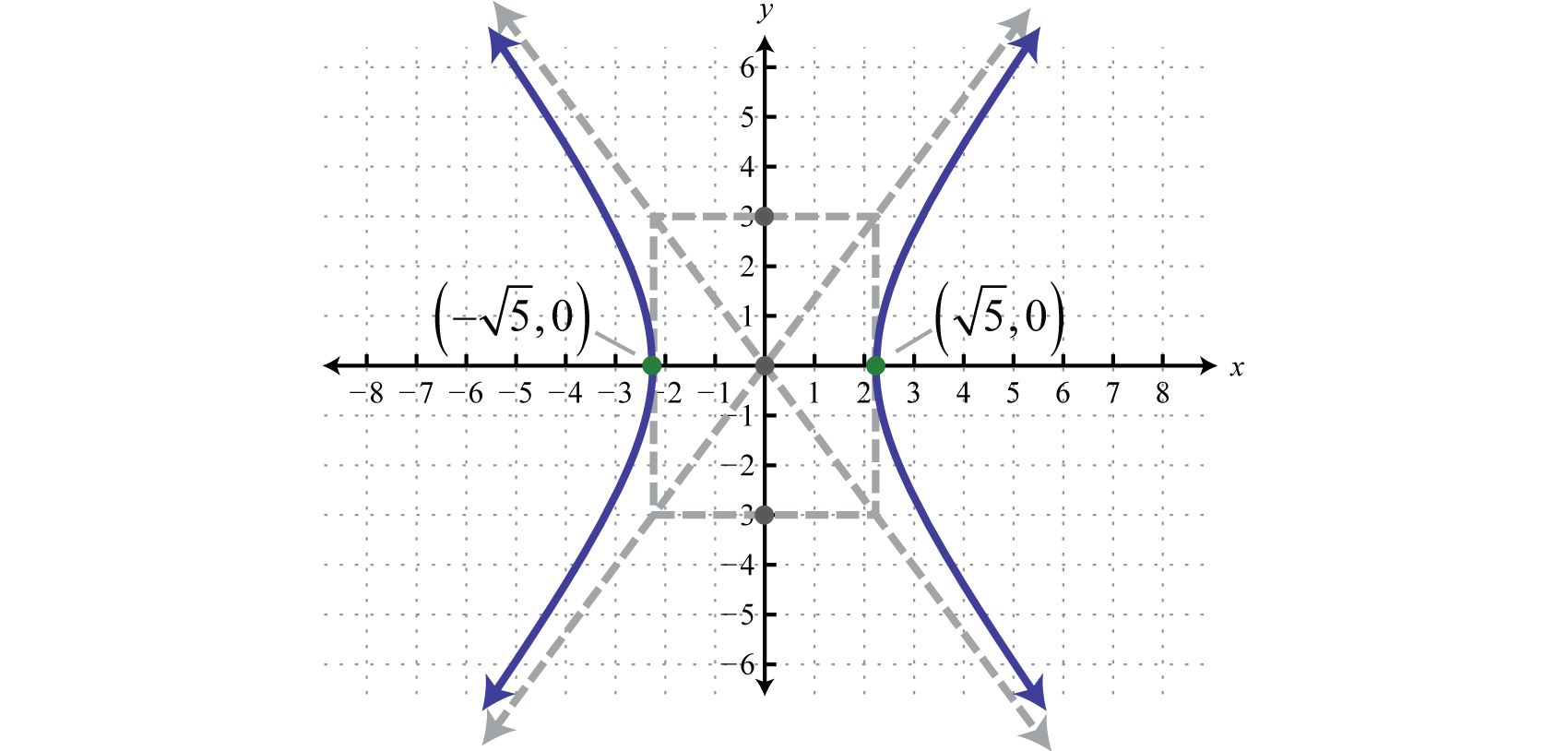



Hyperbolas
X^2y^2=1, (x2)^2 (y1)^2=4 WolframAlpha Volume of a cylinder?X y P(x 1, y 1) P′(x 1 a, y 1 b) Q′(x 2 a, y 2 b) Q(x 2, y 2) A transformation is a function that moves or changes a fi gure in some way to produce a new fi gure called an image Another name for the original fi gure is the preimage The points on the preimage are the inputs for the transformation, and the points on the image areAlgebra questions and answers;
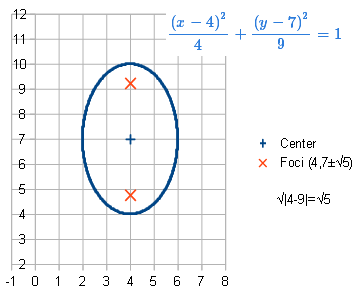



What Does X 4 2 4 Y 7 2 9 1 Represent Socratic




How Do You Find The Center Vertices Foci And Asymptotes Of 4x 2 Y 2 24x 4y 28 0 Homeworklib
Answer (1 of 3) It's the equation of sphere The general equation of sphere looks like (xx_0)^2(yy_0)^2(zz_0)^2=a^2 Where (x_0,y_0,z_0) is the centre of the circle and a is the radious of the circle It's graph looks like Credits This 3D Graph is Which is the graph of x^2/9 y^2/4 = 1 Get the answers you need, now! You will move right and left 2 units from center to find the vertices This comes from √4 that is the denominator of the x2 term Then, go up and down 3 units ( √9) to find corners of a "box" that will create asymptotes for your shape The slopes of the asymptotes will be ± 3 2 for these reasons This graph was created in TInspire, with a template for graphing the conic




Content The Concept Of A Function



How To Construct The Graph Of X 2 Y 2 9 Quora
Plane x = 1 2 The trace in the x = 1 2 plane is the hyperbola y2 9 z2 4 = 1, shown below For problems 1415, sketch the indicated region 14 The region bounded below by z = p x 2 y and bounded above by z = 2 x2 y2 15 The region bounded below by 2z = x2 y2 and bounded above by z = y 7Free graphing calculator instantly graphs your math problemsAlgebra Graph (x^2)/16 (y^2)/9=1 x2 16 y2 9 = 1 x 2 16 y 2 9 = 1 Simplify each term in the equation in order to set the right side equal to 1 1 The standard form of an ellipse or hyperbola requires the right side of the equation be 1 1 x2 16 y2 9



Ellipses And Hyperbolae




Solve Ellipse And Hyperbola Step By Step Math Problem Solver
X 2 − 4x y 2 − 2y = 11 then that is the equation of a circle The coefficients of x 2 and y 2 are 1 And the number must be greater than the negative of the sum of the squares of half the coefficients of x and y Problem 13 Show that the following is the equation of a circle Name the radius and the coördinates of the center x 2 6xCollege Algebra Problems With Answers sample 10 Equation of Hyperbola College algebra problems on the equations of hyperbolas are presented Detailed solutions are at the bottom of the page Problem 1 Find the transverse axis, the center, the foci and the vertices of the hyperbola whose equation is x 2 / 4 y 2 / 9 = 1 Problem 2For a function g (x) = \sqrt {9 x^2}, we can square it to find g^2 (x) = 9 x^2 Adding x^2 to both sides, we now have g^2 (x) x^2 = 9 Replace g (x) = y to get x^2 y^2 = 9 We know , we can square it to find g2(x) = 9− x2 Adding x2 to both sides, we now have g2(x)x2 = 9



Pplato Basic Mathematics Quadratic Functions And Their Graphs
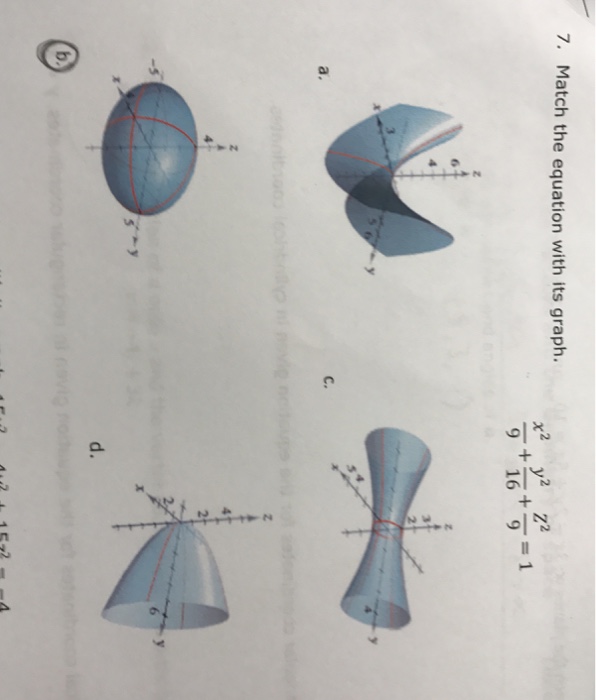



Solved Match The Equation With Its Graph X 2 9 Y 2 16 Chegg Com
In the twodimensional coordinate plane, the equation x 2 y 2 = 9 x 2 y 2 = 9 describes a circle centered at the origin with radius 3 3 In threedimensional space, this same equation represents a surface Imagine copies of a circle stacked on top of each other centered on the zaxis (Figure 275), forming a hollow tubeGraph y=x^24 Find the properties of the given parabola Tap for more steps Rewrite the equation in vertex form Tap for more steps Complete the square for The focus of a parabola can be found by adding to the ycoordinate if the parabola opens upFind the slope and y intercept of 3 xy=2 Graph the line using this information First write 3 xy=2 in the slopeintercept form, y=mxb, by solving for y, getting 3 xy=2 This result shows that the Slope is m=3 and the y intercept is b=− 2 To draw the graph, first locate the y intercept



Solution Graph X 3 2 Y 1 2 4 Y 2 2 4 4 1 X 1 X 2 2 9 Y 3 2 4 1 X 2 2 4 Y 3 2 9 1 Thanks Soo Much




Equation Of An Ellipse In Standard Form And How It Relates To The Graph Of The Ellipse
Solve the system of equations by graphing y = x 2 y 3= I 6 First graph each line, then place a dot indicating the solution to the system 5 4 3 2 3 4 5 5 4Compute answers using Wolfram's breakthrough technology & knowledgebase, relied on by millions of students & professionals For math, science, nutrition, historyGiven the system xy 2z = 9 2 4y 32 = 1 3x 6y 52 = 0 B (a) Find the matrices A and B such that AX = B (b) Use GAUSSIAN elimination to find A1 (c) Solve for x, y, z using this inverse This question hasn't been solved yet Ask an expert Ask an expert Ask an expert done loading




7 04 Hyperbolas
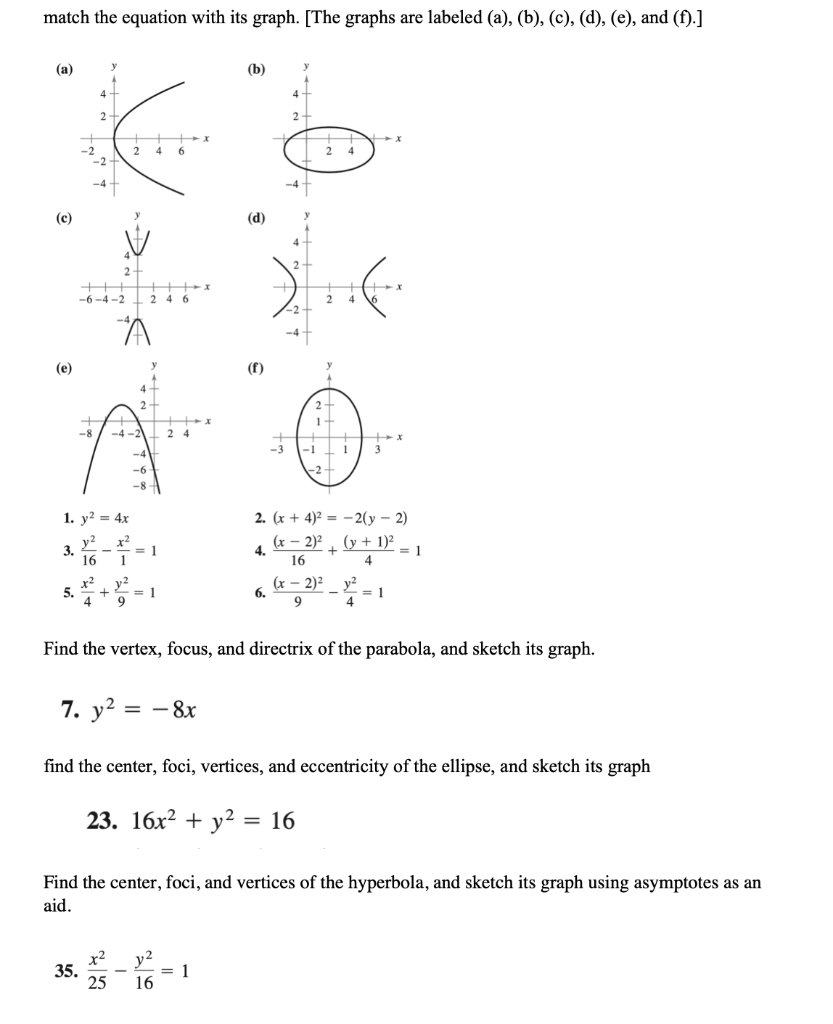



Solved Match The Equation With Its Graph The Graphs Are Chegg Com




Graph X 4 2 4 Y 2 9 1 Brainly Com




Sketch The Graph Of The Following Ellipse X 5 2 4 Y 2 2 9 1 Study Com
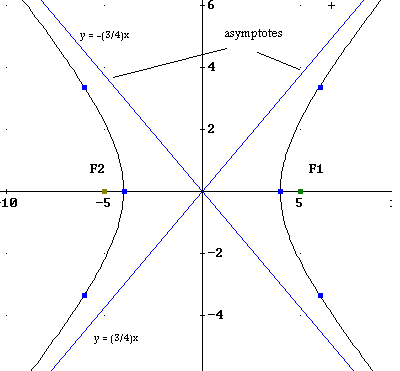



Equation Of Hyperbola Graphing Problems
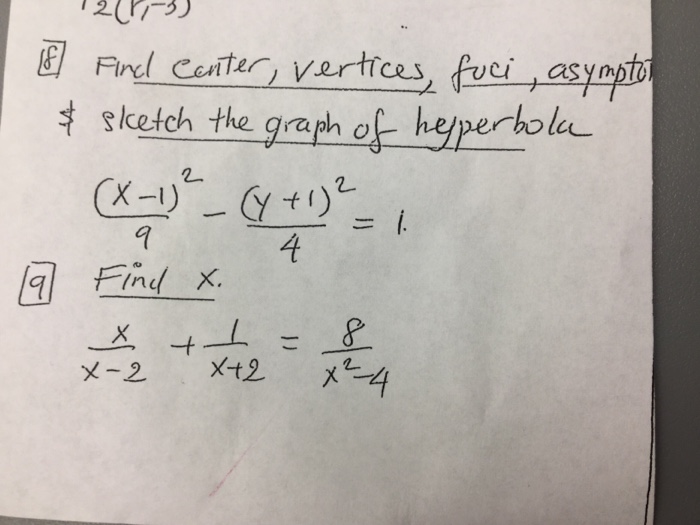



Solved Find Vertices Sletch The Graph Of Chegg Com




1 Consider The Following Equations Y X 2 4 Y X 5 X 0 X 2 Sketch And Shade The Region Bounded By The Graphs Of The Functions
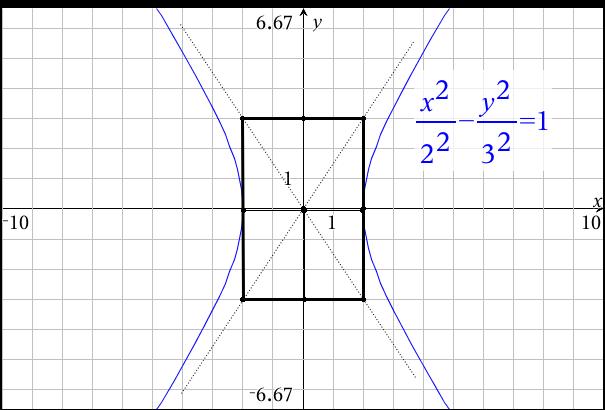



How Do You Graph X 2 4 Y 2 9 1 Socratic



Solved Give The Coordinatesof The Foci Vertices And Convertices Of The Ellipse With Equation X 2 169y 2 25 1 Sketch The Graph And Include Thes Course Hero
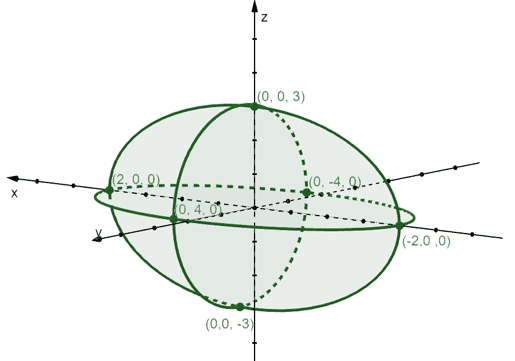



Quadric Surfaces Definition Types And Examples



12 6 Quadric Surfaces Mathematics Libretexts




For Eachof The Following Differential Equations Draw A Itprospt
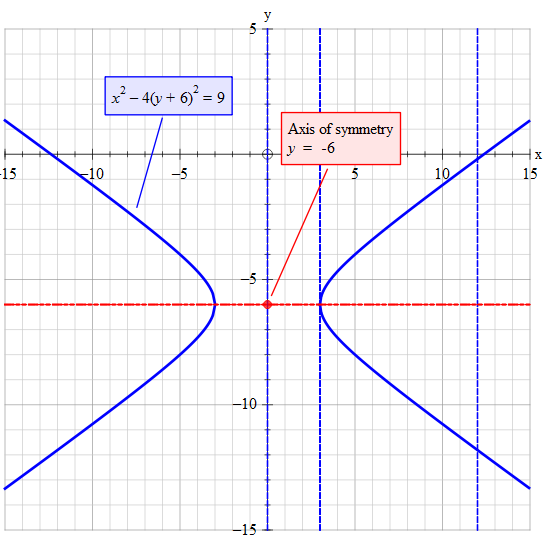



How Do You Graph X 2 4 Y 6 2 9 Socratic
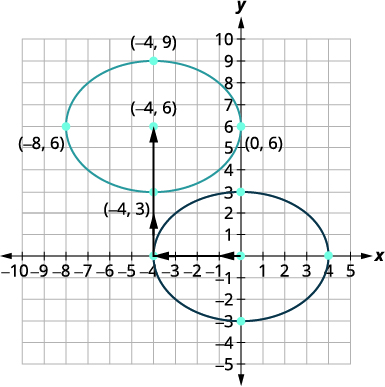



11 4 Ellipses Mathematics Libretexts
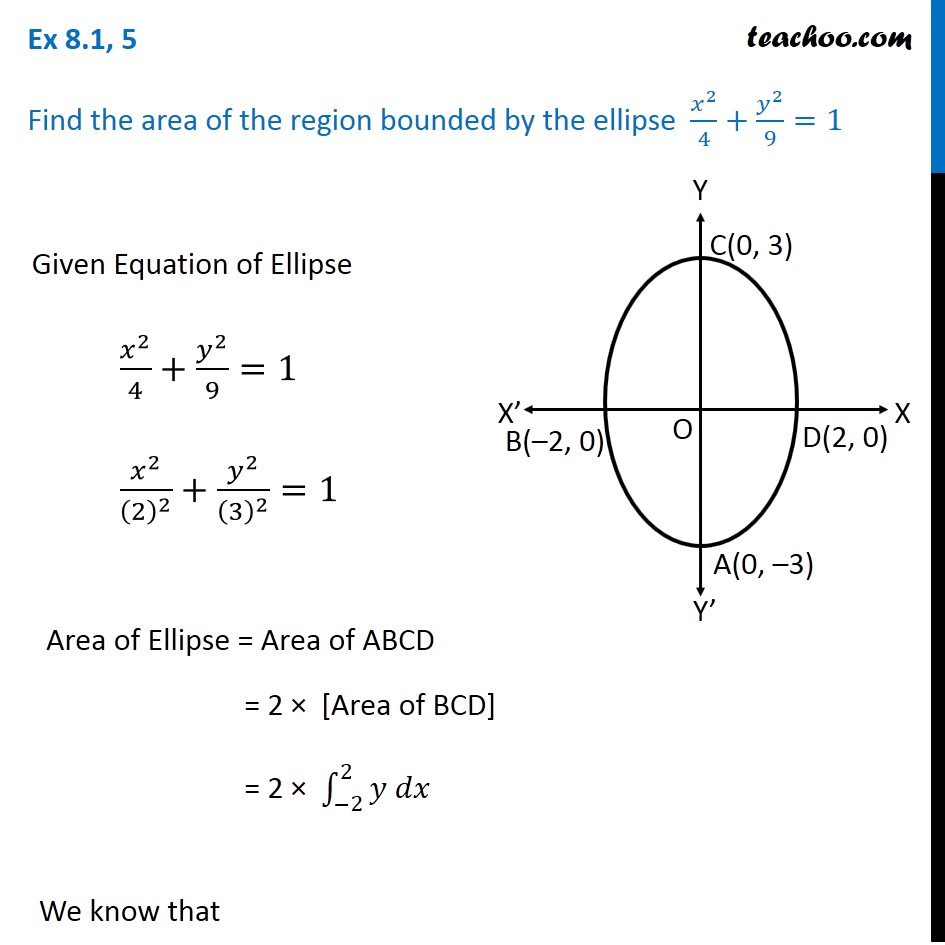



Ex 8 1 5 Find Area By Ellipse X2 4 Y2 9 1 Class 12



14 1 Conic Sections Graphing Calculator By Mathlab User Manual
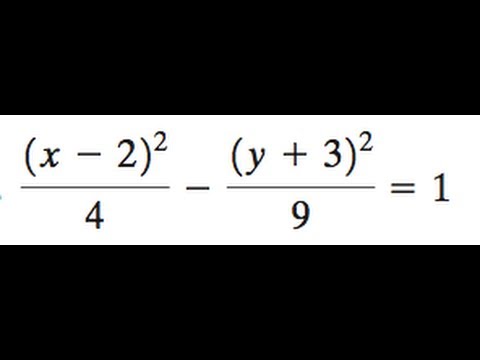



X 2 2 4 Y 3 2 9 1 Hyperbola Youtube




Get Answer The Equation X 2 4 Y 2 9 1 Represents The Graph Of An Ellipse Transtutors



Ellipse Equation




Draw A Rough Sketch Of The Graph Of The Curve X 2 4 Y 2 9 1 And Evaluate The Area Of The Region Under The Curve And Above The X Axis
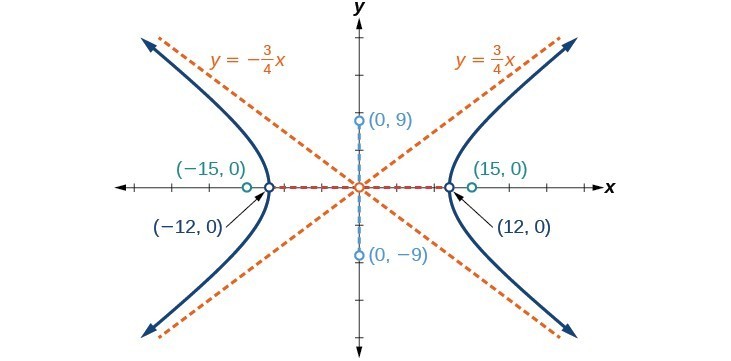



Graph Hyperbolas College Algebra
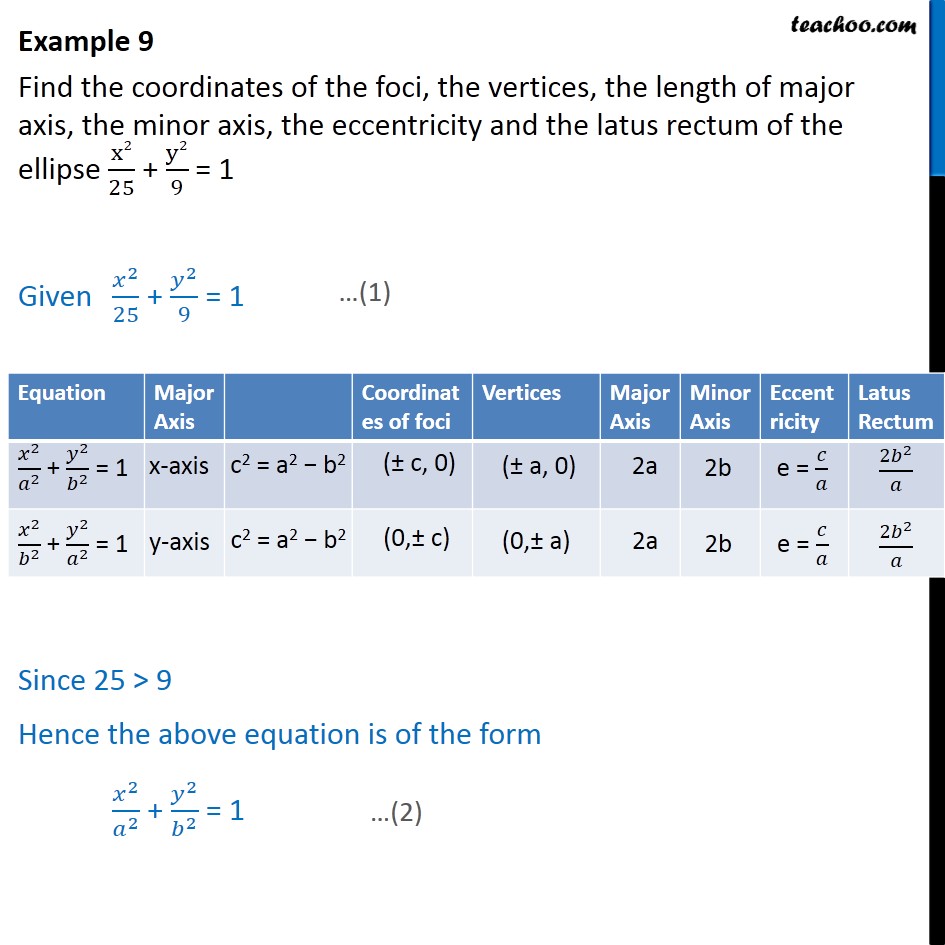



Example 9 X2 25 Y2 9 1 Find Foci Vertices Eccentricity



Which Equation Represents The Graph Frac X24 Fra Gauthmath
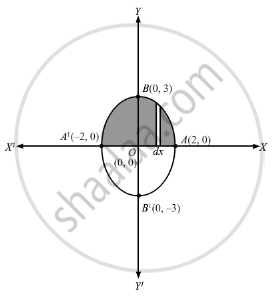



Draw A Rough Sketch Of The Graph Of The Curve X 2 4 Y 2 9 1 And Evaluate The Area Of The Region Under The Curve And Above The X Axis Mathematics Shaalaa Com




Graphing Hyperbola With The Equation X 2 9 Y 2 4 1 Youtube



Solution Find The Vertices And Foci Of The Hyperbola Draw The Graph Y 2 25 X 2 21 1 X 2 9 Y 2 16 1
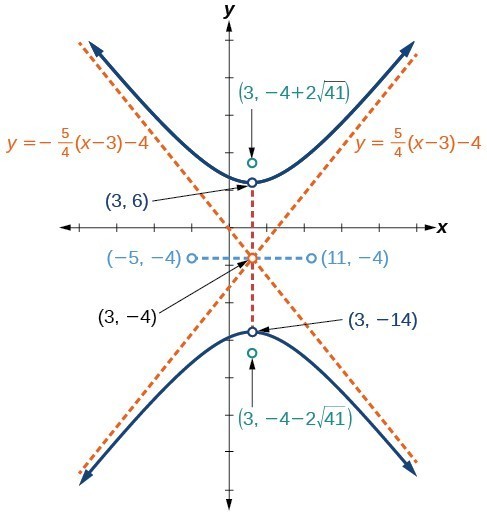



Graph Hyperbolas College Algebra
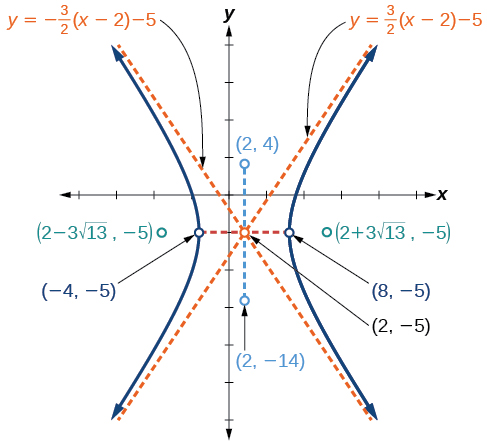



The Hyperbola Precalculus



Find The Area Of The Region Bounded By The Ellipse X 2 4 Y 2 9 1 Sarthaks Econnect Largest Online Education Community



Hyperbola X 2 25 Y 2 9 1 Youtube



Area Of Ellipse Integral



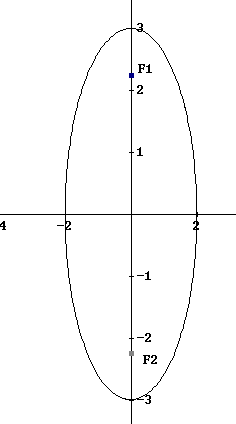
Solution Find The Foci Of The Graph Of X 2 4 Y 2 9 1 Graph The Ellipse



Ellipses And Hyperbolae




7 03 Ellipses And Circles



Equation Of Ellipse Problems
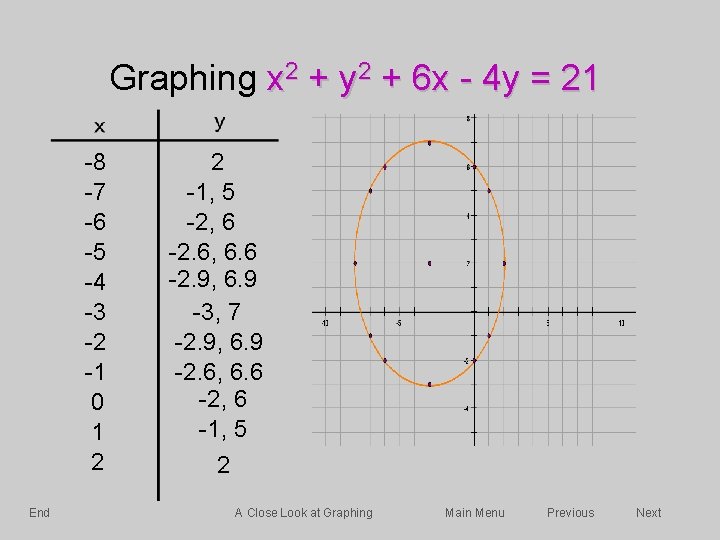



Skip Introduction A Closer Look At Graphing The




Write The Equation Of The Ellipse Shown In The Graph A X 1 2 4 Y 2 2 9 1b X 1 2 9 Brainly Com




What Is The Graph Of Y 2 16 X 2 9 1 Brainly Com




Skip Introduction A Closer Look At Graphing The
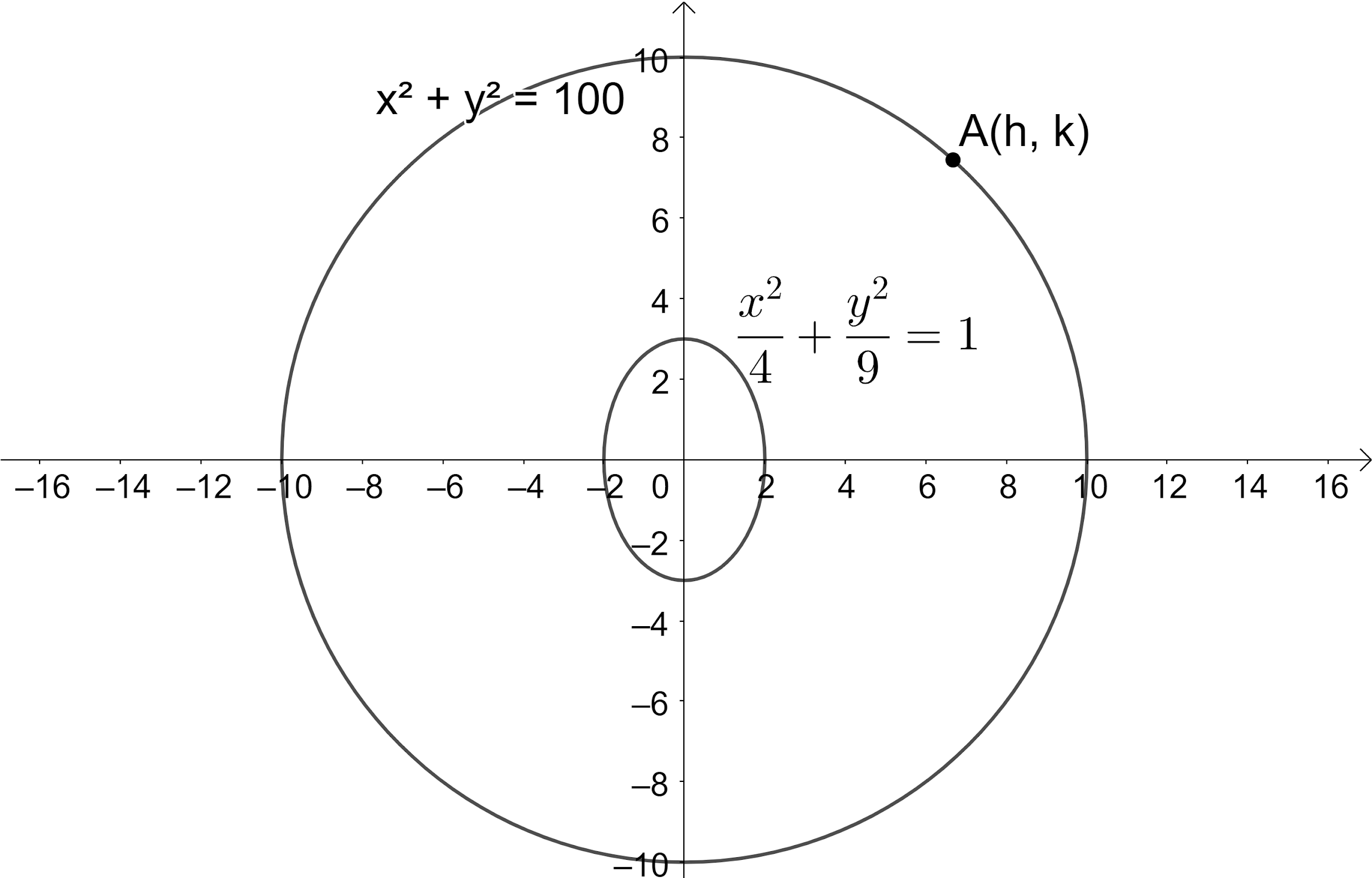



Let The Chord Of Contact Is Drawn From Every Point Class 11 Maths Cbse



コンプリート X 2 4 Y 2 9 1 Graph X 2 4 Y 2 9 1 Graph Nyosspixfcm5




Find The Equation Of The Graph Show Your Work A X 2 4 Y 3 2 9 1 B X 2 4 Y 3 2 9 1 C X 2 9 Y 3 2 4 1 D X 2 16 Y 3 2 81 1 E X 2 16 Y 3 2 81 1 F X 2 4 Y 3 2 9 1 Study Com




Vertices Direction Of A Hyperbola Example 2 Video Khan Academy



Green Globs And Graphing Equations Buffalo State College Flip Ebook Pages 1 6 Anyflip Anyflip



Solution Graph The Ellipse And Its Foci X 2 9 Y 2 4 1



Hyperbola3 Html




Ellipses
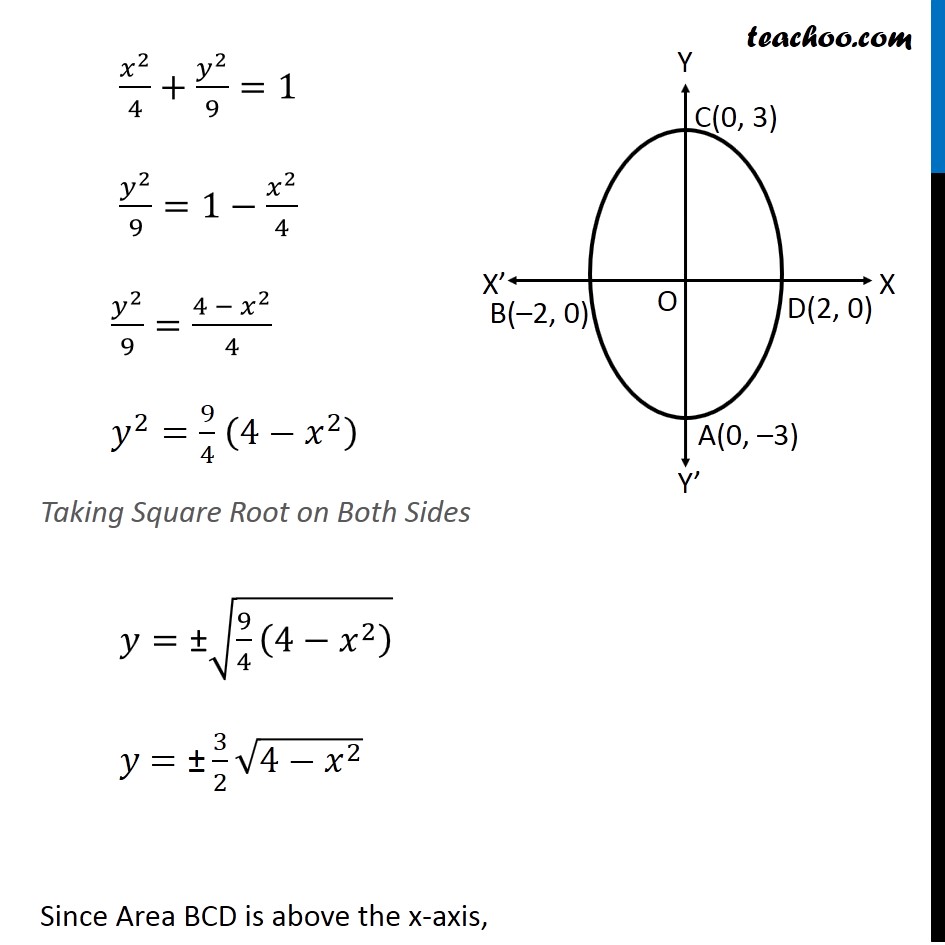



Ex 8 1 5 Find Area By Ellipse X2 4 Y2 9 1 Class 12




Which Is The Graph Of X 2 9 Y 2 4 1 Brainly Com



Digital Lesson Graphs Of Equations The Graph Of



The Smaller Region Bounded By The Ellipse X 2 16 Y 2 9 1 And A Straight Line 3x 4y 12 What Should Be The Area Of That Smaller Region Quora



How Do You Identify If The Equation 9x2 4y2360 Is A Class 12 Maths Cbse
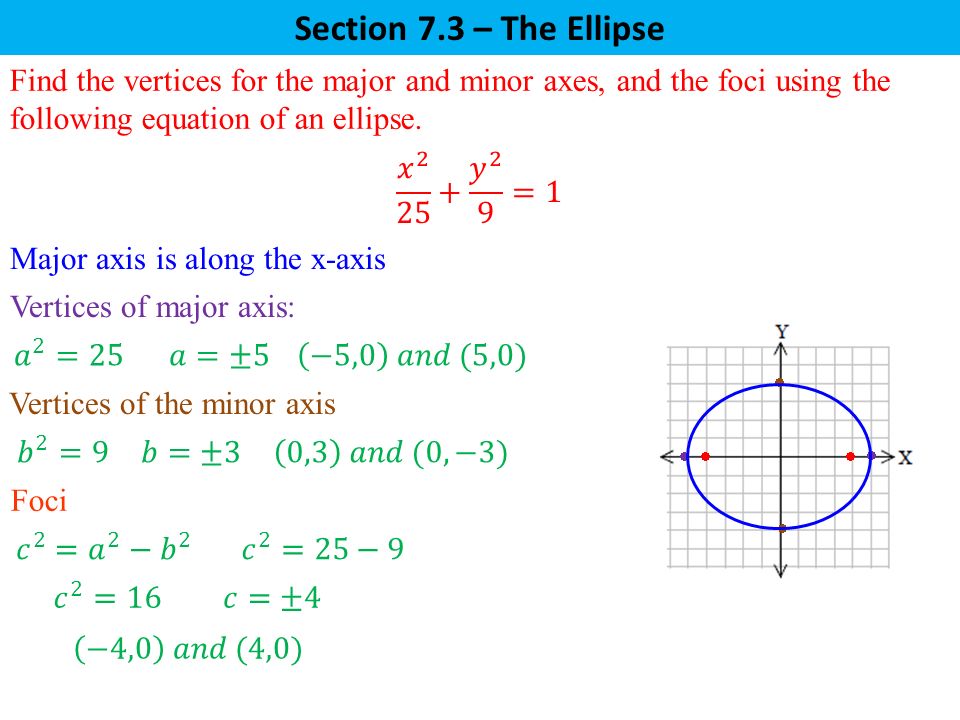



Section 7 3 The Ellipse Ellipse A Set Of Points In A Plane Whose Distances From Two Fixed Points Is A Constant Ppt Download



Jessamine K12 Ky Us




Example 1 Graph The Equation Of A Translated Circle Graph X 2 2 Y 3 2 9 Solution Step 1 Compare The Given Equation To The Standard Form Of Ppt Download



X 4 2 9 Y 2 2 4 1 For The Ellipse Find The Center Foci And Vertices Graph The Equation Youtube
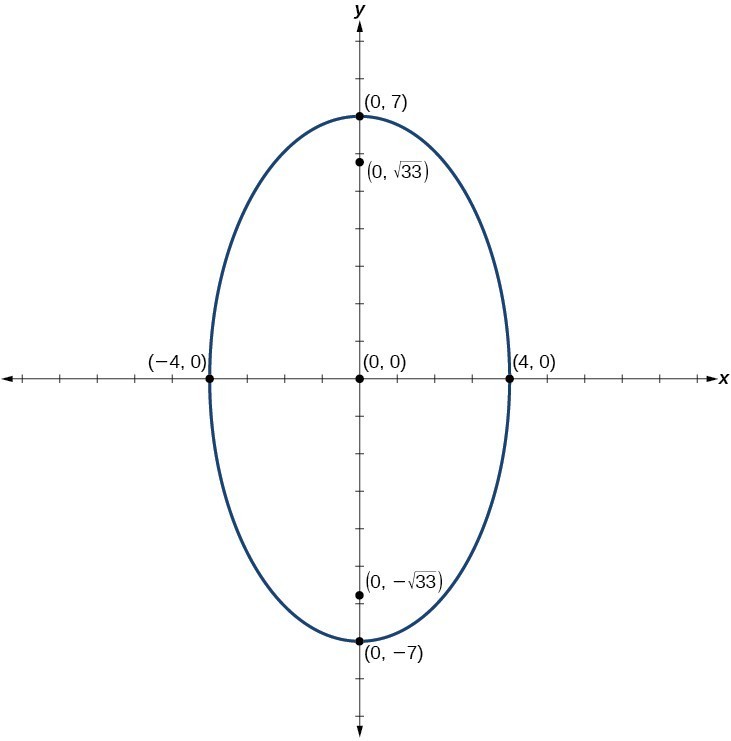



Graphs Of Ellipses College Algebra



Math Drexel Edu
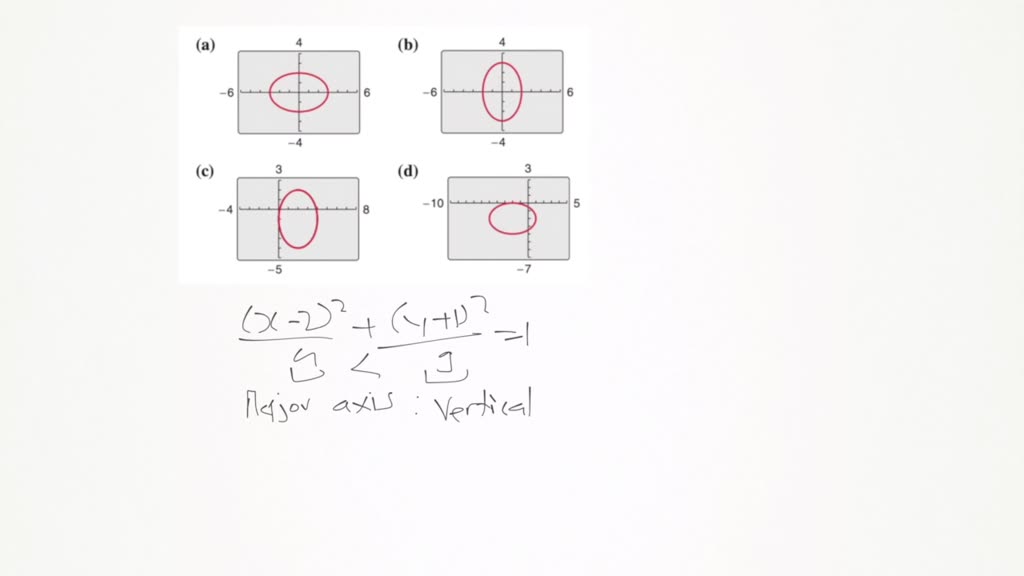



Solved Match The Equation With Its Graph The Graphs Are Labeled A B C And D Frac X 2 2 4 Frac Y 1 2 9 1




Content The Concept Of A Function




Graph Equations System Of Equations With Step By Step Math Problem Solver



Hyperbola3 Html



Green Globs And Graphing Equations Buffalo State College Flip Ebook Pages 1 6 Anyflip Anyflip



Solved 1 Exercises In Exercises 1 8 Graph The Ellipse Find The Center The Lines Which Contain The Major And Minor Axes The Vertices The Endp Course Hero



Graph Y X 7 X 3 Mathskey Com
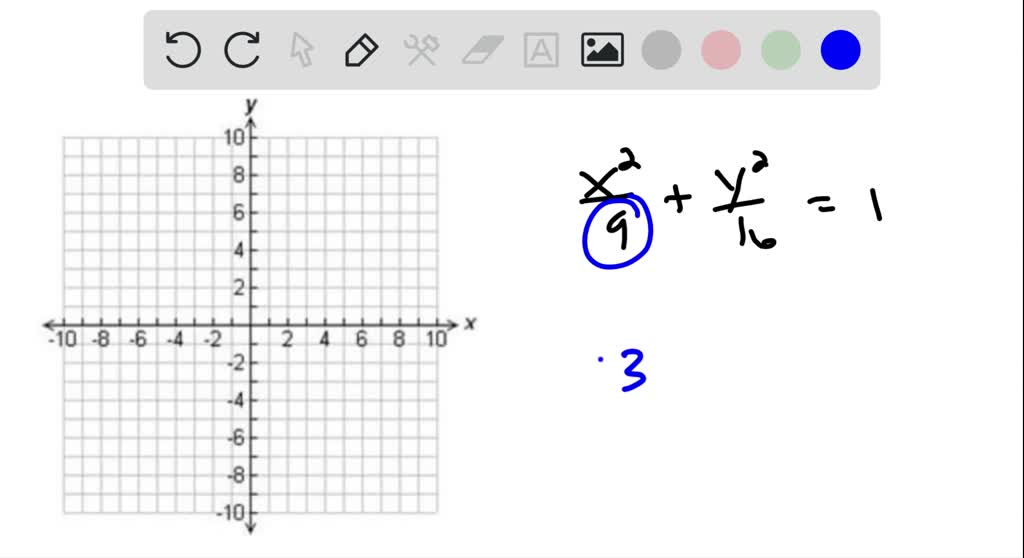



Solved Graph Each Ellipse Frac X 2 2 16 Frac Y 1 2 9 1
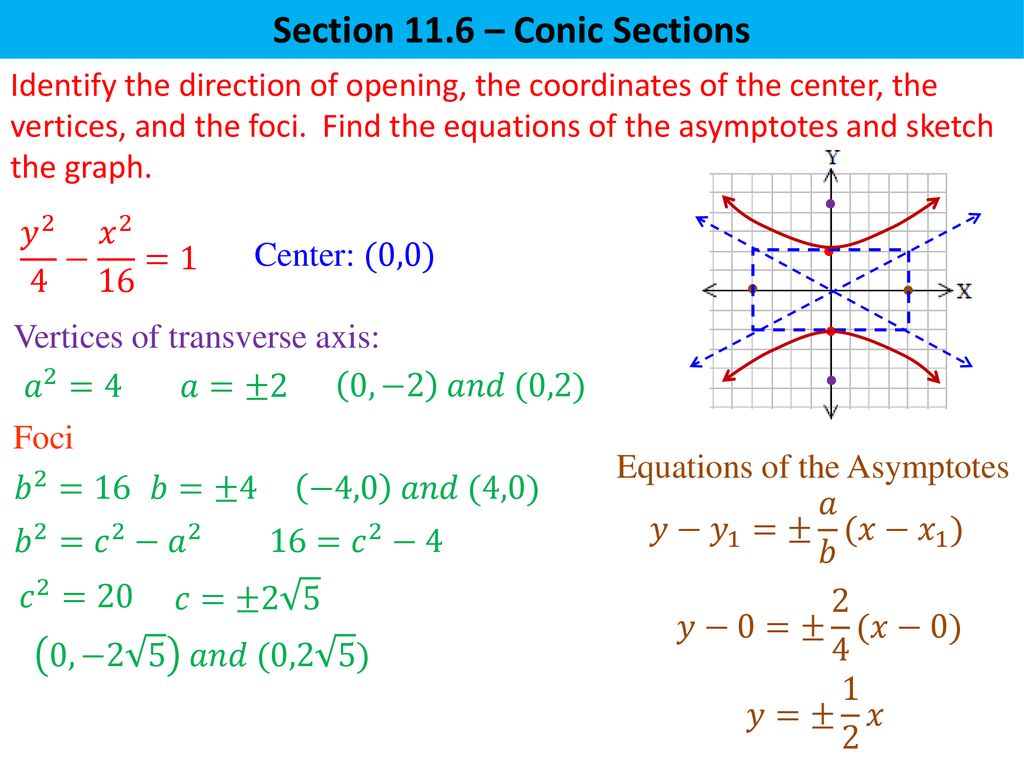



Section 11 6 Conic Sections Ppt Download



1



Math Drexel Edu




Maple Essentials Section 6 More On Graphing Application Center



Pslv Egs Circles




Solved For The Surface X 2 4 Y 2 9 Z 2 16 1 Give The Chegg Com




Draw A Rough Sketch Of The Graph Of The Curve X 2 4 Y 2 9 1 And Evaluate The Area Of The Region Under The Curve And Above The X Axis




5 1 Indentifying Linear Equations



The Foci Of Hyperbola 9x 2 16y 2 18x 32y 151 0 Are




How Do You Find The Foci And Sketch The Hyperbola X 2 9 Y 2 4 1 Socratic


